Synthesis collecting#
Wherein I e-collect -- and attempt to comprehend -- inspiring instances of synthesis.
System entropy à la Information Theory#

Excerpting the application of Claude Shannon's work to thermodynamics here: the information entropy of a system is the amount of "missing" information needed to determine a particular microstate, given the macrostate -- so a continuum from least information, maybe the absense of information, to complete information. Shannon, whose sights were on electronic communications at the time, was focusing on expressing mathematically the "informational value" of a communicated message in a channel, the study of which is seen as the beginning of Information Theory. It became clear the synthesis was equally applicable to thermodynamic entropy.
Claude Shannon, Bell Labs Engineer, IAS fellow, MIT faculty member (theory developed in 1940s)
Maxwell et al. - Electromagnetic spectrum#
Might be the mother of all syntheses: a host of science legends are part of this story, from those making the precursor discoveries through to those who validated and expanded from Maxwell's synthesizing/unifying electromagnetic field equations -- Herschel, Ørsted, Faraday, Hertz, and Röntgen among them.
Maxwell's equations and analysis predicted the possibility and behavior of waves in the field, that those theoretical waves must travel at about the known speed of light, and that an infinite range of frequencies were implied. Hence, the spectrum of electromagnetic waves, a tiny fraction of which are visible to humans, and another small fraction of which provides the strata of radio communications (the realm ushered in by Hertz's incisive application and analysis of Maxwell's formulas).
As is often quoted, when asked if he stood on the shoulders of Newton, Albert Einstein replied "No, on the shoulders of Maxwell".
An alternative, astronomy-themed visual clipped from a display at the Very Large Array walking tour:

Attractors in dynamic systems#
The attactor can be said to be the state of equilibrium that is inherent to a given dynamic system. The dynamic system is described by a mathematical function that receives a set of inputs or readings that capture the state of the system at a given instance in time. Even for a system we might intuitively expect to exhibit random or chaotic behavior over time (the system of virus-spreading through a population, maybe), the corresponding function describing the system can often enough be fairly short and simple. And, given it has a function in the first place, one would expect that however unusual or complex the result may be it cannot in fact be random.
When one iterates on the function -- by taking the function's output points and feeding them back to the function as the inputs for the next iteration -- a shape emerges and fills in progressively after a high quantity of iterations have been run. The shape represents the system's equilibrium in the form of a "basin of attraction" where the results of any iteration will fall -- this is what practitioners refer to as the system's "attractor".
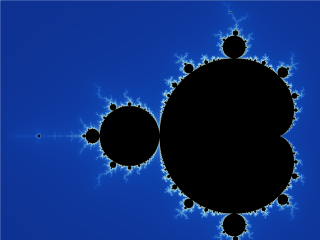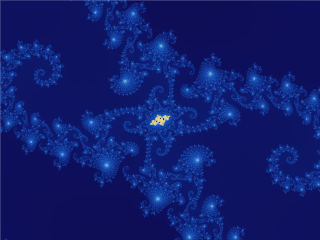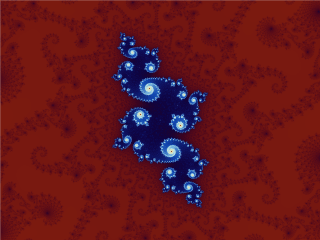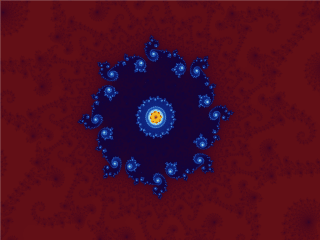
For the dynamic systems in which the equilibrium/basin of attraction manifests in a fractal shape, their attactor is deemed a "Strange Attractor" -- presumably because the fractal shape is a fairly strange/complex shape for the attractor to take compared to the alternatives (in some systems the attractor can be a fixed point or a simple oscillation).
In 1963, MIT mathematician and meteorologist Edward Lorenz arrived at perhaps the most famous strange attactor -- a fractal evoking the shape of a butterfly, as it were -- as he studied the property of convection in the Earth's atmosphere (a dynamic system by anyone's standards) from an information-theory lens. The butterfly shape inspired the exploration of the famed "butterfly effect" in this research, wherein iteration demonstrated inputs altered by something as subtle as a butterfly's wings flapping could notably alter the iteration-to-iteration system dynamics. (Note, however, that even in these iterations, the inputs, impacted though they are by the butterfly's beating wings, are still simply processed by the mathematical function, with the result falling, "non strangely", upon the so-called basin of attaction.)
Visual representations of fractals are often wondrous in themselves, of course, so it should be no surprise to find oneself having something of a "music of the cosmos" moment to see a fractal depicting the landscape of a complex dynamic system in the realm of chaos theory.
Partial list of sources:
https://www.wondriumdaily.com/order-in-randomness-fractals-and-chaotic-systems/
https://softologyblog.wordpress.com/2017/03/04/2d-strange-attractors/
https://en.wikipedia.org/wiki/Phase_space
https://www.sciencedirect.com/topics/physics-and-astronomy/strange-attractor
https://www.chaos-math.org/en/chaos-vii-strange-attractors.html
https://www.allthescience.org/what-is-a-strange-attractor.htm
https://uwaterloo.ca/applied-mathematics/future-undergraduates/what-you-can-learn-applied-mathematics/dynamical-systems/strange-attractors